How to Teach Adding and Subtracting Fractions with Unlike Denominators

 

Looking for how to introduce and teach adding and subtracting fractions with unlike denominators?

Let’s talk about using manipulatives, pictures, number lines, and equations. In addition, I’m sharing how to differentiate instruction for students when they add and subtract fractions with unlike denominators.

What to look for in this post:

• The progression of the standards for adding and subtracting fractions with unlike denominators
• Introducing adding and subtracting fractions with unlike denominators
• Using manipulatives to work with fractions
• Representing adding and subtracting fractions with unlike denominators
• Abstract: Using equations to add and subtract fractions with unlike denominators
• The milestones of adding and subtracting fractions with unlike denominators
• Differentiation for adding and subtracting fractions with unlike denominators

If you prefer to hear me talk through this, here is a video featuring all of this content:

[0:28] The progression of the standards for adding and subtracting fractions with unlike denominators

For most states that base their standards off the common core standards, third grade students begin with basic fractions. They work with equivalent fractions and identifying fractions. In fourth grade, most states have students begin adding and subtracting fractions and mixed numbers with like denominators. In fifth grade, they begin adding and subtracting fractions with like denominators.

[1:09] Introducing adding and subtracting fractions with unlike denominators

When introducing this concept to students, we want to take a research-based approach. The best approach for teaching mathematics is the CRA method: Concrete, Representational, and Abstract. Concrete methods involve giving students opportunities to get their hands on manipulatives. Representational methods allow students to represent these hands-on experiences through pictures, number lines, or other visual models. Abstract methods include working with equations or algorithms. There are no hands-on or visual components to these methods.

For adding and subtracting fractions with unlike denominators, concrete methods involve using pattern blocks and fraction bars in a way that supports students’ understanding of the concept of changing to common denominators. In a representational model, we are going to use pictures and number lines. For abstract methods, we are going to use written equations.

As you work through the CRA model with your students, it is important to give them enough time to work through each approach. Some students may need more time with hands-on activities, while others may be able to jump very quickly into representational methods. I would not do all three of these in the same lesson, as one builds upon the other. We want to give students a strong foundation first.

[3:15] Using manipulatives to work with fractions

My favorite manipulatives to use when working with fractions are fraction bars or fraction tiles. If you do not have these available, pattern blocks can be a good alternative. I spoke more about these manipulatives in a previous post about adding and subtracting fractions with like denominators. 

If you watched the video or read the post, you know that I don’t begin by talking about fractions. Instead, I begin by talking about everyday items. For example, I have one eraser and I add a paperclip - what do I have? After a couple creative responses, they will come up with: we have one eraser and one paperclip, which is exactly what we want them to say.

We are going to tell them that, with fractions, we can change them and combine them even though they are not the same. So the first thing I want to do is work with our fraction bars as a concrete method for learning to add fractions with unlike denominators. I like students to keep one whole visible for reference. Then I ask something like, what is one third plus one sixth? Remember the paperclip and the eraser. We are talking about two different things, but we need to keep something about them the same.

Give them a moment to play around with that, and hopefully they have already had experience with equivalent fractions. If so, they should be able to come up with something that gives them the same distance or the same amount. Instead of thinking about the question as one third plus one sixth, we can rename it as two sixths plus one sixth. In that case, we know we have three sixths. This is a quick win, and then we can work on getting things down to simplest form. You can challenge them to give you an equivalent fraction for three sixths, using as few tiles as possible.

We can then have them think about one third minus one sixth. For some students, it might be helpful to leave the one third up for students to see. Sometimes for subtraction, I have them stack equivalent fractions on top of one another so they can see what it looks like to take away one sixth. Other times I will have them trade out. We discuss and agree that two sixths is the same as one third, so we can swap it out.

With pattern blocks, we are a bit more limited because we only have access to halves, thirds, and sixths. I want them to recognize that we cannot have a paperclip and an eraser - we need them to be the same. We can trade out, as a red piece is the same as three greens, and see that one half plus one sixth is the same as four sixths.

If we want to subtract, we have to make sure that students do not add to their pieces. I ask them to make all their pieces red or greens, having students do fair trades.

[14:46] Representing adding and subtracting fractions with unlike denominators

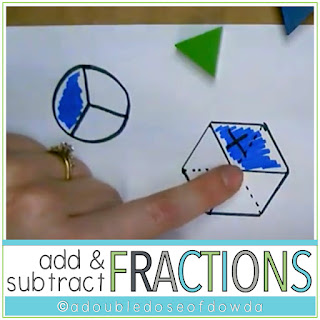
The next piece is to talk about how we would represent these concepts. Using pictures, we could draw circles and color in the given fractions. Rather than adding on one sixth, we want the students to realize that they need to turn the sixths into thirds. I like to have students draw dotted lines so we can take away the sixth pieces and put an X over the top to see what we have left. What I really love about this strategy is how it takes students from the concrete to the representational.

Another representational strategy is to use a number line. Students can really struggle with drawing their own number lines, so if you have fraction bars you can have them make number lines based on those. Using one whole as the number line, you can then help them to notice where one half plus one sixth would go. You can help them to re-number their number lines: ⅙, 2/6, 3/6, 4/6, ⅚, and 6/6.

Once they get the hang of this, I may ask them a challenge question: In as few pieces as possible, what is a simpler way to write this? Some students will be able to go straight to understanding that 4/6 is the same as ⅔ because they understand equivalent fractions. Other students can stack their ⅓ and their other ⅓ to see that it is ⅔.

You can also help them to use their number lines to subtract fractions with unlike denominators. If we gave them one half minus one sixth, we would have them go through the same process and then make the jump backwards to two sixths. Again, challenge time: who can make it in as few pieces as possible?

[22:53] Abstract: Using equations to add and subtract fractions with unlike denominators

If we write out ⅓ + ⅙, we can remind students of our paperclips and erasers. One third and one sixth cannot be combined as they are written, so we need to change them into something that has the same denominator for both fractions. This is where you have a conversation with them about least common multiples. I like to have students list the multiples of three and the multiples of six. Having them list them out on their paper tends to produce better results, because it makes them slow down a bit rather than rushing through the problem.

So, I show them how to do this:

1 x 3 = 3

2 x 3 = 6

3 x 3 = 9

4 x 3 = 12

5 x 3 = 15

I tell them to do a few at a time, and then I tell them to do the sixes:

1 x 6 = 6

2 x 6 = 12

3 x 6 = 18

4 x 6 = 24

5 x 6 = 30

Then I will ask them which ones we have in common. They both have 12 and 6, so I will circle those. Then I will tell them we want the smallest one possible, because as mathematicians we can be a little lazy. Sometimes we want the quickest and easiest way to do something. So six fits the bill in this case, and we need to set up equivalent fractions with six as the denominator.

Students have to understand that we are not looking to change the values of either fraction. We still need one third plus one sixth. So our one sixth can stay the same, but we need our one third to become something equivalent in sixths: three times two is six, so it becomes two sixths. We multiply the numerator and the denominator by two. Then we can leave the denominator, and just add the numerators: 2/6 + ⅙ = 3/6.

[27:26] The milestones of adding and subtracting fractions with unlike denominators

For any new concept, I like to identify the milestones - the new learning students need to work through in order to complete these problems. This helps me to pinpoint where students might be having trouble.

The first milestone is that they have to be able to identify the least common multiples of the denominators so that they can make the least common denominator.

The second milestone is that they need to know how to make equivalent fractions. We have to make sure they can convert fractions to equivalent fractions that have the same denominators.

The third milestone is that they can add and subtract just the numerators of the fractions.

Lastly, students need to be able to put their answers in simplest form.

[28:29] Differentiation for adding and subtracting fractions with unlike denominators

What if students are having trouble? Keeping those milestones in mind will help you to understand where students may need support. Even before this concept of adding and subtracting fractions with unlike denominators, some students may need assistance with third or fourth grade math concepts. They may need to review basic fraction concepts, such as adding and subtracting fractions with like denominators or equivalent fractions.

If you have students who need a bit of a challenge, you can help them to come up with sums greater than one whole. If you are using smaller fractions for the class, you can have these students use larger fractions or throw in mixed numbers if they are ready. You can also have them add or subtract three or more fractions.

Looking for more resources for teaching how to add and subtract fractions with unlike denominators?

When it comes to practicing these skills, I have put together a bundle of some of my favorite activities for adding and subtracting fractions with unlike denominators. It includes seven resources:

• Two matching games
• Two BINGO games
• Two worksheets
• One anchor chart

Students will enjoy these activities and they will ask to do them over and over. The matching games include three levels: below level, on level, and a challenge level that includes word problems. The anchor chart can be used as a one-page handout, or you can print it as half pages for interactive notebooks or poster versions.

The Activities Bundle is available on Teachers Pay Teachers.

You can also grab this free download, which is a teaching guide including everything we talked about in this post. It is a one-page sheet that gives you all this information so that you will be prepared to teach adding and subtracting fractions with unlike denominators.

Check out these related posts:
How to Teach Adding and Subtracting Fractions with Like Denominators
How to Teach Metric Measurement for Third Grade
How to Teach Metric Conversions for Fourth and Fifth Grades

Check out these related YouTube videos:
How to Teach Adding and Subtracting Fractions with Like Denominators
How to Teach Adding and Subtracting Mixed Numbers
How to Teach Third Grade Measurement
How to Teach Converting Measurements for Fourth and Fifth Grades
How to Teach Polygons and Quadrilaterals for Third Grade